Assessment & Rote Learning: Math Conundrums

Multiply Two Negatives and You Get a Positive.

So, I take ‘Rote Learning’ and multiply that by ‘Teaching to the Test’ and that equals ‘Better Standardized Test Results’? Well, that’s not exactly what this post is about… but this is a good lead in. And for those of you that don’t know why -3 x -4 = +12, I do provide a link that might help.

In some ways I think this really should be two posts, one on Assessment and one on Rote Learning of Multiplication/Division Skills, but I also think they fit well together.

---

I’m in the process of marking some Algebra tests.

Just so you know, 7 x 4 = 32 and 6 x 8 = 52.

I just want that on the record.

In both cases the student’s algebra was correct.

In fact, in both those cases the error made the algebra much more difficult, with the variable becoming a fraction rather than in integer.

So, how would you evaluate these two questions?

---

Assessment

Before you read this, Dan Meyer’s How Math Must Assess, and his linked mini-thesis are worthy reads.

I remember doing a Math/Assessment Pro-D at the start of a Staff Meeting a few years ago. I gave everyone a Fraction Quiz and an answer key for a fictitious student. The quiz was out of 20. (I have the questions, but need to track down the answers I created to add to this post.)

The first question asked the student to reduce fractions to lowest terms, (4 fractions for 4 marks - they included 2 proper fractions, & 2 improper, one of which reduced to a whole number).

The second question said, “Solve. Put all answers in lowest terms. (2 marks each)”. There were a total of 8 questions, 2 each for adding, subtracting, multiplying and dividing fractions.

The student made one consistent error when reducing.

Staff members had many questions including, “Can I give half marks?” -All of which I answered, “You are marking the quiz, you decide.”

On the low end, one teacher who had never taught Math gave the student 8/20 - I think he gave 1 mark each for getting the first parts of the 2nd question correct. On the other end of the spectrum, our LAC (Learning Assistance Center) teacher gave the student 18/20. All other teachers varied within these two scores, with no single score being an obvious favorite.

To be honest, the quiz answers were very contrived, and I doubt a student would make such an error so consistently without making others, but the point was well taken.

What are we assessing? Is our assessment measuring what we say it is? Are we assessing the right things?

---

Rote Learning (for Multiplication Tables… and Related Division Questions)
If you teach Math, here is a New Voice (#6 of 7). I stumbled on to Amanda Waye’s Understanding Multiplication blog doing a Google Search for this post. Her Opposing Views on Teaching Methods has made this post easy for me… read her post and I can get down to the ‘nitty gritty’ without a whole lot of background details.

Rote learning. I know the opposing arguments. I even agree with them as I will demonstrate later. But when a kid arrives in my class in Grade 8 and doesn’t know their times tables it drives me crazy… When they can’t multiply 4 x 7, or can’t see that 7/28 can be reduced… I have to wonder… how can I meaningfully teach them integers or algebra?

Now, I’m neither suggesting that students sit at one table and memorize another table for hours on end; Nor am I suggesting that rote learning is a singular approach to learning multiplication. But in order to get students to be more numerate, we need not have the pendulum swing completely away from drilling some basics.

Multiplication is repeated addition, it is about adding ‘groups of‘ a number. It is a simple concept.

If a student just has rote comprehension of their multiplication tables in their early years, it will help them more than it could possibly hurt them. For those of you that had multiplication drilled into them, did it scar you? Are you wounded by it? If so, I would argue that it was a result of poor delivery, not the actual memorization. I know that I memorized my tables, but as an adult I have no recollection of the process… just as I don’t remember learning to read. Furthermore, as a Social Studies trained, Arts Degree student, I know that a strong foundation in basic skills helped make my transition to teaching Math a lot easier than if I had lacked such a strong background.

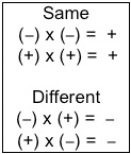
Here is the crux of my point: When you have a solid understanding of Math fundamentals, it is easy to build new, more challenging concepts on to your base knowledge.

Example: When multiplying integers I teach the ‘rules’, the algorithm, but I also teach ‘Why?’. A student who has rote understanding of their times tables will see within my Multiplying Integers lessons that multiplication is repeated addition… a student lacking basic multiplication skills usually cannot go beyond the ‘rules’ since the multiplication itself is a neuron-taxing challenge to them.

---

You need an understanding of basic skills before you can move on to more challenging tasks.

You can’t teach a skateboarder to do a Ollie when they still have issues staying on their skateboard… They need to be competent on their skateboard- without thinking about their balance, timing etc. before they learn the more complicated moves. Once a skater has the fundamentals of an Ollie within their repertoire, they have the foundation to perform even more skills/tricks.

Integers and Algebra both build on a foundation knowledge of multiplication skills.

Use rote memorization, flash-cards, games online… make it fun… do a song and dance, stand on your head… but what ever you do, don’t let your kids get to Grade 8 without knowing their multiplication tables!

- - - - -

Image of Skater: IMG_8459 by Outside the Box on Flickr

Originally posted: March 23rd, 2007 Reflection upon re-reading and re-posting: I think my last paragraph is a bit over the top, but I still feel strongly about the ideas in this post. Our move to numeracy: right-brained/more spacial/conceptual/problem based learning is great, but cannot be done in isolation from a strong foundation of basic facts. Now that I am out of the classroom, I think I can be a little more objective about my own practice as a Math teacher. (It also helps that I am giving learning support to two different teachers in Math as well.) I simply was not constructivist enough! Sometimes, I justified teaching the rule first by claiming that it appeased students and allowed them to relax and learn the ‘why’ afterwards… was I appeasing students or myself? That said, Basic Math Facts are the foundation or scaffolding that support conceptual understanding of Mathematics. Any learning constructed in a Math class will crumble without basic support structures in place. Multiplication (and related division) tables are an essential base for success!

Update: May 1st/08 Darren Kuropatwa attempted to post a comment and it ‘borked’, so he posted it instead. I asked if I could share it here too… I couldn’t agree with him more!

Breathtaking post, or was it three? Assessment I did the same exercise with my dept. We also had the same vastly differing results you did. At a provincial in-service about 9 or 10 years back I participated in the same exercise using real student generated work. Results varied from around 33% to 80%. This is one facet of f Academe’s Dirty Little Secret. Anyway, in my dept. we’ve been looking at how we assess all the content in all the courses we teach; one course at a time, one unit at a time. We’re trying to develop a consistent approach to assessment at least within our building. We’ll be “at it” for a while yet. Basic Skills Fluent knowledge and recall of basic addition, subtraction, multiplication and division facts are essential for ANY student to experience success in math. I’m on the same page you are Dave. A grade 9 student, who struggles (mightily) with her multiplication facts, and I were talking about this last week. As I was trying to help her I asked why she thinks I feel it so important for her to become fluent in her recall of the multiplication table: “I know, I know, some day I might not have a calculator and I might need to multiply two numbers.” [Oy! Who tells kids this stuff? And do they really believe that? — I mean the adults, not the kids. I know the kids don’t believe that.] “No. That’s not why. You’ll always be able to get a calculator if you need to multiply a bunch of numbers. That’s not the reason. It’s that you need to know the language of math so you can join the conversation.” “If your teacher is trying to teach you why multiplying pairs of negative numbers always have a positive result, or why, when we divide fractions, we ‘multiply by the reciprocal’ they’re going to talk about stuff like 7×8 and assume you know it’s 56 and go on to discuss some deeper ideas. If you’re hung up on 7×8, need to pull out a calculator, you’re going to miss the entire conversation. Your brain will be back 5 steps while everyone else is talking about this other stuff. By the time you figure out what’s going on you won’t know what’s going on. You’ll feel lost and confused and fall farther behind.” “Why do I need to know math anyway?” “For the same reason you need to know how to read. Because it’s a fundamental way that humans communicate with each other and understand the world around them. If you can read but you can’t understand mathematics then there will be giant tracts of things happening in the world around you that you’ll never understand.” [Whew! Went on a bit of a rant there. I’m going to get a cup of tea … Cheers Dave!] Darren Kuropatwa